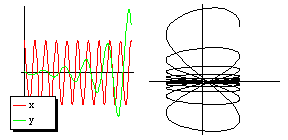
You can see the presentation
slides shown on October 3, 2001,
at the Third
Turin Fortnight on Nonlinear Analysis.
[home]
Here is a short mathematical paper presented at Prof. Gary Meisters's retirement conference in May, 1997, available in electronic format only (if you have trouble downloading from the States you can try the copy I downloaded to Udine). There is also an attached Mathematica notebook (updated May 7, 2000, with a correction) with implementation of the algorithm. In the same site you can find a picture of myself during the lecture.
Joint work with Prof. Gaetano Zampieri (University of Torino, Dept. of Mathematics), Time Reversibility and Energy Conservation for Lagrangian Systems with Nonlinear Nonholonomic Constraints, Reports on Mathematical Physics, vol. 45, issue 2, (2000), pages 217-227.
Abstract. When is a nonholonomic Lagrangian system time-reversible? We prove that a simple sufficient condition is that (skipping over some minor technicalities) both the Lagrangian L(t,q,p) and the set of the triples (t,q,p) that satisfy the constraints are invariant by exchange of (t,p) into (-t,-p). Another question is: when is energy conserved in a nonholonomic autonomous Lagrangian system? A likewise easy sufficient condition is that the set of the couples (q,p) satisfying the constraints is a cone with respect to p (meaning that if (q,p) is admissible then (q,rp) is admissible too for all positive r). Time-reversibility and energy conservation are independent properties, in the sense that neither one implies the other. Both properties hold at the same time for any autonomous system with a “natural” Lagrangian and with constraints that are homogenous in p.
Sorry, this is not available for download. See however the item in Mathematical Reviews.
Joint work with Dr. Halszka Tutaj-Gasinska (Jagiellonian University, Dept. of Mathematics, Krakóv, Poland), On the Span Invariant for Cubic Similarity, Annales Polonici Mathematici LXXVI.1-2 (2001), 113-119. Proceedings of the conference Poly99 conference.
Abstract. Let A be a real n-times-n matrix and x an n-ple of real numbers. Define F(x) as the n-tuple of 3rd powers of the components of Ax. We have some conjectures and some partial results about the span of F, that is, the smallest linear space containing the range of F. This is of some interest for the Jacobian conjecture.
Joint work with Prof. Angelo Barone-Netto and Prof. Mauro de Oliveira Cesar (University of São Paulo, Dept. of Mathematics and Statistics, Brasil), Explicit Criteria for the Stability, Dipartimento di Matematica e Informatica, Università di Udine, Rapporto di Ricerca 7/2000/RR. Accepted for publication on the Journal of Differential Equations.
Abstract. Consider the system of differential equationsx''=-xf(x), y''=-yg(x), where f(0)>0, g(0)>0. We give an abstract result that characterizes the couples of functions f,g for which the origin is a stable equilibrium for the system. We then develop explicit either necessary or sufficient conditions for stability or instability in terms of the values of f,g and their first two derivatives at the origin.

You can see the presentation
slides shown on October 3, 2001,
at the Third
Turin Fortnight on Nonlinear Analysis.
Locally Convex Homogenous Multifunctions and their Conjugates. Dipartimento di Matematica e Informatica, Università di Udine, Rapporto di Ricerca 8/2000/RR.
Abstract. A locally convex real function defined on a nonconvex set may not have a global convex conjugate function (or Fenchel transform). In this paper we introduce a concept of locally convex multifunction that allows us to talk of conjugation of any locally convex (multi)function. For simplicity the theory is restricted to the homogeneous (multi)functions in dimension 2. Several examples are given.
This page has been accessed
times since July, 2001.